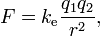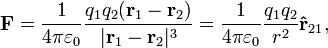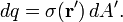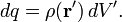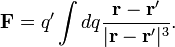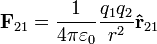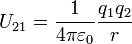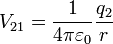Coulomb's law
Background to the schools Wikipedia
SOS believes education gives a better chance in life to children in the developing world too. To compare sponsorship charities this is the best sponsorship link.
| Electromagnetism |
|---|
 |
|
Electrostatics
|
|
Magnetostatics
|
|
Electrodynamics
|
|
Electrical network
|
|
Covariant formulation
Electromagnetic tensor
( stress–energy tensor)
|
Coulomb's law, sometimes called the Coulomb law, is an equation describing the electrostatic force between electric charges. It was developed in the 1780s by French physicist Charles Augustin de Coulomb and was essential to the development of the theory of electromagnetism. Coulomb's law may be stated in scalar form as follows:
- The magnitude of the electrostatic force between two point electric charges is directly proportional to the product of the magnitudes of each of the charges and inversely proportional to the square of the total distance between the two charges.
Scalar form
The scalar form of Coulomb's law will only describe the magnitude of the electrostatic force between two electric charges. If direction is required, then the vector form is required as well. The magnitude of the electrostatic force (F) on a charge (q1) due to the presence of a second charge (q2), is given by
where r is the distance between the two charges and ke a proportionality constant. A positive force implies a repulsive interaction, while a negative force implies an attractive interaction.
The proportionality constant ke, called Coulomb's constant is related to the properties of space and can be calculated exactly:
In SI units the speed of light in vacuum, denoted c0 is defined as 299,792,458 m· s−1, and the magnetic constant (μ0), is defined as 4π × 10−7 H· m−1, leading to the definition for the electric constant (ε0) as ε0 = 1/(μ0c2
0) ≈ 8.854187817×10−12 F· m−1. In cgs units, the unit charge, esu of charge or statcoulomb, is defined so that this Coulomb force constant is 1.
This formula says that the magnitude of the force is directly proportional to the magnitude of the charges of each object and inversely proportional to the square of the distance between them. The exponent in Coulomb's Law has been found to differ from −2 by less than one in a billion.
When measured in units that people commonly use (such as SI—see International System of Units), the electrostatic force constant (ke) is numerically much much larger than the universal gravitational constant (G). This means that for objects with charge that is of the order of a unit charge (C) and mass of the order of a unit mass (kg), the electrostatic forces will be so much larger than the gravitational forces that the latter force can be ignored. This is not the case when Planck units are used and both charge and mass are of the order of the unit charge and unit mass. However, charged elementary particles have mass that is far less than the Planck mass while their charge is about the Planck charge so that, again, gravitational forces can be ignored. For example, the electrostatic force between an electron and a proton, which constitute a hydrogen atom, is almost 40 orders of magnitude greater than the gravitational force between them.
Coulomb's law can also be interpreted in terms of atomic units with the force expressed in Hartrees per Bohr radius, the charge in terms of the elementary charge, and the distances in terms of the Bohr radius.
Electric field
It follows from the Lorentz Force Law that the magnitude of the electric field (E) created by a single point charge (q) at a certain distance (r) is given by:
For a positive charge, the direction of the electric field points along lines directed radially away from the location of the point charge, while the direction is the opposite for a negative charge. The SI units of electric field are volts per meter or newtons per coulomb.
Vector form
In order to obtain both the magnitude and direction of the force on a charge, 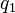 at position
at position 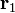 , experiencing a field due to the presence of another charge, q2 at position
, experiencing a field due to the presence of another charge, q2 at position 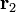 , the full vector form of Coulomb's law is required.
, the full vector form of Coulomb's law is required.
where  is the separation of the two charges. Note that this is simply the scalar definition of Coulomb's law with the direction given by the unit vector,
is the separation of the two charges. Note that this is simply the scalar definition of Coulomb's law with the direction given by the unit vector, 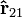 , parallel with the line from charge
, parallel with the line from charge 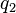 to charge
to charge 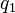 .
.
If both charges have the same sign (like charges) then the product 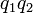 is positive and the direction of the force on
is positive and the direction of the force on 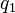 is given by
is given by 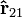 ; the charges repel each other. If the charges have opposite signs then the product
; the charges repel each other. If the charges have opposite signs then the product 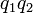 is negative and the direction of the force on
is negative and the direction of the force on 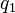 is given by
is given by 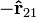 ; the charges attract each other.
; the charges attract each other.
System of discrete charges
The principle of linear superposition may be used to calculate the force on a small test charge, 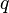 , due to a system of
, due to a system of 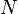 discrete charges:
discrete charges:
where 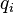 and
and  are the magnitude and position respectively of the
are the magnitude and position respectively of the 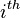 charge,
charge, 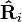 is a unit vector in the direction of
is a unit vector in the direction of 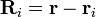 (a vector pointing from charge
(a vector pointing from charge 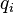 to charge
to charge 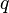 ), and
), and 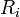 is the magnitude of
is the magnitude of 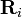 (the separation between charges
(the separation between charges 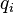 and
and 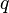 ).
).
Continuous charge distribution
For a charge distribution an integral over the region containing the charge is equivalent to an infinite summation, treating each infinitesimal element of space as a point charge 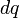 .
.
For a linear charge distribution (a good approximation for charge in a wire) where 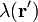 gives the charge per unit length at position
gives the charge per unit length at position 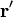 , and
, and 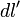 is an infinitesimal element of length,
is an infinitesimal element of length,
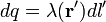 .
.
For a surface charge distribution (a good approximation for charge on a plate in a parallel plate capacitor) where 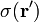 gives the charge per unit area at position
gives the charge per unit area at position 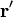 , and
, and 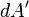 is an infinitesimal element of area,
is an infinitesimal element of area,
For a volume charge distribution (such as charge within a bulk metal) where 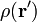 gives the charge per unit volume at position
gives the charge per unit volume at position 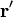 , and
, and  is an infinitesimal element of volume,
is an infinitesimal element of volume,
The force on a small test charge 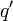 at position
at position 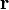 is given by
is given by
Graphical representation
Below is a graphical representation of Coulomb's law, when  . The vector
. The vector 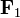 is the force experienced by
is the force experienced by 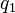 . The vector
. The vector 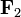 is the force experienced by
is the force experienced by 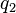 . Their magnitudes will always be equal. The vector
. Their magnitudes will always be equal. The vector 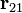 is the displacement vector between two charges (
is the displacement vector between two charges (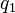 and
and 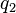 ).
).
Electrostatic approximation
In either formulation, Coulomb's law is fully accurate only when the objects are stationary, and remains approximately correct only for slow movement. These conditions are collectively known as the electrostatic approximation. When movement takes place, magnetic fields are produced which alter the force on the two objects. The magnetic interaction between moving charges may be thought of as a manifestation of the force from the electrostatic field but with Einstein's theory of relativity taken into consideration.
Table of derived quantities
| Particle property | Relationship | Field property | |||||
| Vector quantity |
|
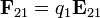 |
|
||||
| Relationship | 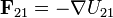 |
 |
|||||
| Scalar quantity |
|
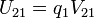 |
|