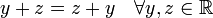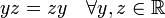Commutative property
About this schools Wikipedia selection
SOS Children made this Wikipedia selection alongside other schools resources. Do you want to know about sponsoring? See www.sponsorachild.org.uk
Commutativity is a widely used mathematical term that refers to the ability to change the order of something without changing the end result. It is a fundamental property in most branches of mathematics and many proofs depend on it. The commutativity of simple operations was for many years implicitly assumed and the property was not given a name or attributed until the 19th century when mathematicians began to formalize the theory of mathematics.
Common uses
The commutative property (or commutative law) is a property associated with binary operations and functions. Similarly, if the commutative property holds for a pair of elements under a certain binary operation then it is said that the two elements commute under that operation.
In group and set theory, many algebraic structures are called commutative when certain operands satisfy the commutative property. In higher branches of math, such as analysis and linear algebra the commutativity of well known operations (such as addition and multiplication on real and complex numbers) is often used (or implicitly assumed) in proofs.
Mathematical definitions
The term "commutative" is used in several related senses.
1. A binary operation ∗ on a set S is said to be commutative if:
- x ∗ y = y ∗ x for every x,y ∈ S
- An operation that does not satisfy the above property is called noncommutative.
2. One says that x commutes with y under ∗ if:
- x ∗ y = y ∗ x
3. A binary function f:A×A → B is said to be commutative if:
- f(x,y) = f(y,x) for every x, y ∈ A.
History and etymology
Records of the implicit use of the commutative property go back to ancient times. The Egyptians used the commutative property of multiplication to simplify computing products. Euclid is known to have assumed the commutative property of multiplication in his book Elements. Formal uses of the commutative property arose in the late 18th and early 19th century when mathematicians began to work on a theory of functions. Today the commutative property is a well known and basic property used in most branches of mathematics. Simple versions of the commutative property are usually taught in beginning mathematics courses.
The first use of the actual term commutative was in a memoir by Francois Servois in 1814, which used the word commutatives when describing functions that have what is now called the commutative property. The word is a combination of the French word commuter meaning "to substitute or switch" and the suffix -ative meaning "tending to" so the word literally means "tending to substitute or switch." The term then appeared in English in Philosophical Transactions of the Royal Society in 1844.
Related properties
Associativity
The associative property is closely related to the commutative property. The associative property states that the order in which operations are performed does not affect the final result. In contrast, the commutative property states that the order of the terms does not affect the final result.
Symmetry
Symmetry can be directly linked to commutativity. When a commutative operator is written as a binary function then the resulting function is symmetric across the line y = x. As an example, if we let a function f represent addition (a commutative operation) so that f(x,y) = x + y then f is a symmetric function which can be seen in the image on the right.
Examples
Commutative operations in everyday life
- Putting your shoes on resembles a commutative operation since it doesn't matter if you put the left or right shoe on first, the end result (having both shoes on), is the same.
- When making change we take advantage of the commutativity of addition. It doesn't matter what order we put the change in, it always adds to the same total.
Commutative operations in math
Two well-known examples of commutative binary operations are:
- The addition of real numbers, which is commutative since
-
- For example 4 + 5 = 5 + 4, since both expressions equal 9.
- The multiplication of real numbers, which is commutative since
-
- For example, 3 × 5 = 5 × 3, since both expressions equal 15.
- Further examples of commutative binary operations include addition and multiplication of complex numbers, addition of vectors, and intersection and union of sets.
Noncommutative operations in everyday life
- Washing and drying your clothes resembles a noncommutative operation, if you dry first and then wash, you get a significantly different result than if you wash first and then dry.
- The Rubik's Cube is noncommutative. For example, twisting the front face clockwise, the top face clockwise and the front face counterclockwise (FUF') does not yield the same result as twisting the front face clockwise, then counterclockwise and finally twisting the top clockwise (FF'U). The twists do not commute. This is studied in group theory.
Noncommutative operations in math
Some noncommutative binary operations are:
- subtraction is noncommutative since

- division is noncommutative since
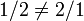
- matrix multiplication is noncommutative since
Mathematical structures and commutativity
- An abelian group is a group whose group operation is commutative.
- A commutative ring is a ring whose multiplication is commutative. (Addition in a ring is by definition always commutative.)
- In a field both addition and multiplication are commutative.
- The centre is the largest commutative subset of a group.