
Riemann surface
About this schools Wikipedia selection
This Schools selection was originally chosen by SOS Children for schools in the developing world without internet access. It is available as a intranet download. SOS Child sponsorship is cool!
In mathematics, particularly in complex analysis, a Riemann surface, first studied by and named after Bernhard Riemann, is a one-dimensional complex manifold. Riemann surfaces can be thought of as "deformed versions" of the complex plane: locally near every point they look like patches of the complex plane, but the global topology can be quite different. For example, they can look like a sphere or a torus or a couple of sheets glued together.
The main point of Riemann surfaces is that holomorphic functions may be defined between them. Riemann surfaces are nowadays considered the natural setting for studying the global behaviour of these functions, especially multi-valued functions such as the square root and other algebraic functions, or the logarithm.
Every Riemann surface is a two-dimensional real analytic manifold (i.e., a surface), but it contains more structure (specifically a complex structure) which is needed for the unambiguous definition of holomorphic functions. A two-dimensional real manifold can be turned into a Riemann surface (usually in several inequivalent ways) if and only if it is orientable. So the sphere and torus admit complex structures, but the Möbius strip, Klein bottle and projective plane do not.
Geometrical facts about Riemann surfaces are as "nice" as possible, and they often provide the intuition and motivation for generalizations to other curves, manifolds or varieties. The Riemann-Roch theorem is a prime example of this influence.
Definitions
There are several equivalent definitions of a Riemann surface.
- A Riemann surface X is a complex manifold of complex dimension one. This means that X is a well-behaved space endowed with a so-called atlas: for every point x ∈ X there is an environment, which looks like the complex plane. The map carrying the structure of the complex plane to the Riemann surface is called chart. Two charts which overlap are required to be compatible in a certain sense.
- A Riemann surface is a Riemannian manifold of (real) dimension two - whence the name Riemann surface together with a conformal structure. Again, manifold means that locally at any point x of X, the space is supposed to be like the real plane. The supplement "Riemann" signifies that X is endowed with a so-called Riemannian metric g, which allows to measure angles on the manifold. Two such metrics are considered equivalent if the angles they measure are the same. Choosing a metric, and hence an equivalence class of metrices on X is the additional datum of the conformal structure.
A complex structure gives rise to a conformal structure by choosing the standard Euclidean metric given on the complex plane and transporting it to X by means of the charts.
Examples
- The complex plane C is perhaps the most basic Riemann surface. The map f(z) = z (the identity map) defines a chart for C, and {f} is an atlas for C. The map g(z) = z* (the conjugate map) also defines a chart on C and {g} is an atlas for C. The charts f and g are not compatible, so this endows C with two distinct Riemann surface structures. In fact, given a Riemann surface X and its atlas A, the conjugate atlas B = {f* : f ∈ A} is never compatible with A, and endows X with a distinct, incompatible Riemann structure.
- In an analogous fashion, every open subset of the complex plane can be viewed as a Riemann surface in a natural way. More generally, every open subset of a Riemann surface is a Riemann surface.
- Let S = C ∪ {∞} and let f(z) = z where z is in S \ {∞} and g(z) = 1 / z where z is in S \ {0} and 1/∞ is defined to be 0. Then f and g are charts, they are compatible, and { f, g } is an atlas for S, making S into a Riemann surface. This particular surface is called the Riemann sphere because it can be interpreted as wrapping the complex plane around the sphere. Unlike the complex plane, it is compact.
- The theory of compact Riemann surfaces can be shown to be equivalent to that of projective algebraic curves that are defined over the complex numbers and non-singular. For example, the torus C/(Z+τ Z), where τ is a complex non-real number, corresponds, via the Weierstrass elliptic function associated to the lattice Z+τ Z, to an elliptic curve given by an equation
-
- y2=x3 + a x + b.
- Tori are the only Riemann surfaces of genus one, surfaces of higher genera g are provided by the hyperelliptic surfaces
- y2=P(x),
- where P is a complex polynomial of degree 2g+1.
- Important examples of non-compact Riemann surfaces are provided by analytic continuation.
Further definitions and properties
As with any map between complex manifolds, a function f: M → N between two Riemann surfaces M and N is called holomorphic if for every chart g in the atlas of M and every chart h in the atlas of N, the map h o f o g-1 is holomorphic (as a function from C to C) wherever it is defined. The composition of two holomorphic maps is holomorphic. The two Riemann surfaces M and N are called biholomorphic (or conformally equivalent to emphasize the conformal point of view) if there exists a bijective holomorphic function from M to N whose inverse is also holomorphic (it turns out that the latter condition is automatic and can therefore be omitted). Two conformally equivalent Riemann surfaces are for all practical purposes identical.
Orientability
We noted in the preamble that all Riemann surfaces, like all complex manifolds, are orientable as a real manifold. The reason is that for complex charts f and g with transition function h = f(g-1(z)) we can consider h as a map from an open set of R2 to R2 whose Jacobian in a point z is just the real linear map given by multiplication by the complex number h'(z). However, the real determinant of multiplication by a complex number α equals |α|2, so the Jacobian of h has positive determinant. Consequently the complex atlas is an oriented atlas.
Functions
Every non-compact Riemann surface admits non-constant holomorphic functions (with values in C). In fact, every non-compact Riemann surface is a Stein manifold.
In contrast, on a compact Riemann surface X every holomorphic function with value in C is constant due to the maximum principle. However, there always exists non-constant meromorphic functions (=holomorphic functions with values in the Riemann sphere C ∪ {∞}). More precisely, the function field of X is a finite extension of C(t), the function field in one variable, i.e. any two meromorphic functions are algebraically dependent. This statement generalizes to higher dimensions, see Siegel (1955).
Analytic vs. algebraic
The above fact about existence of nonconstant meromorphic functions can be used to show that any compact Riemann surface is a projective variety, i.e. can be given by polynomial equations inside a projective space. Actually, it can be shown that every compact Riemann surface can be embedded into the complex projective plane. This is a surprising theorem: Riemann surfaces are given by locally patching charts. If one global condition, namely compacity, is added, the surface is necessarily algebraic. This feature of Riemann surfaces allows to study them with either the means of analytic or algebraic geometry. The corresponding statement for higher-dimensional objects is false, i.e. there are compact complex 2-folds which are not algebraic. On the other hand, every projective complex manifold is necessarily algebraic, see Chow's theorem.
As an example, consider the torus T := C/(Z+τ Z). The Weierstrass function 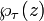 belonging to the lattice Z+τ Z is a meromorphic function on T. This function and its derivative
belonging to the lattice Z+τ Z is a meromorphic function on T. This function and its derivative  generate the function field of T. There is an equation
generate the function field of T. There is an equation
where the coefficients g2 and g3 depend on τ, thus giving an elliptic curve Eτ in the sense of algebraic geometry. Reversing this is accomplished by the j-invariant j(E), which can be used to determine τ and hence a torus.
Classification of Riemann surfaces
The realm of Riemann surfaces can be divided into three regimes: hyperbolic, parabolic and elliptic Riemann surfaces. This distinction is given by the uniformization theorem which states that every simply connected Riemann surface is conformally equivalent one of the following:
- the complex plane C
- the Riemann sphere C ∪ {∞}, also denoted P1C
or
- the open disk D := {z ∈ C : |z| < 1} or equivalently the upper half-plane H := {z ∈ C : Im(z) > 0}.
According to the equivalence of two definitions given above, the uniformization theorem can also be stated in terms of conformal geometry: every connected Riemann surface X admits a unique complete 2-dimensional real Riemann metric with constant curvature -1, 0 or 1 inducing the same conformal structure. The surface X is called hyperbolic, parabolic, and elliptic, respectively. The existence of these three types parallels the several (Non-)Euclidean geometries.
The general technique of associating a manifold X its universal cover Y, and expressing the original X as the quotient of Y by the group of deck transformations gives a first overview over Riemann surfaces.
Elliptic Riemann surfaces
By definition, these are the surfaces X with constant curvature +1. The Riemann sphere C ∪ {∞} is the only example. (Elliptic curves are examples of parabolic Riemann surfaces. The naming comes from the history: elliptic curves are associated to elliptic functions, which in turn show up in calculating the circumference of ellipses).
Parabolic Riemann surfaces
By definition, these are the surfaces X with constant curvature 0. Equivalently, by the uniformization theorem, the universal cover of X has to be the complex plane.
There are then three possibilities for X. It can be the plane itself, an annulus, or a torus
- T := C / (Z ⊕ τZ).
The set of representatives of the cosets are called fundamental domains. Care must be taken insofar as two tori are always homeomorphic, but in general not biholomorphic to each other. This is the first appearance of the problem of moduli. The modulus of a torus can be captured by a single complex number τ with positive imaginary part. In fact, the marked moduli space ( Teichmüller space) of the torus is biholomorphic to upper half-plane or equivalently the open unit disk.
Hyperbolic Riemann surfaces
The Riemann surfaces with curvature -1 are called hyperbolic. This group is the "biggest" one.
The celebrated Riemann Mapping Theorem states that any simply connected strict subset of the complex plane is biholomorphic to the unit disk. Therefore the open disk with the Poincaré-metric of constant curvature -1 is the local model of any hyperbolic Riemann surface. According to the uniformization theorem above, all hyperbolic surfaces are quotients of the unit disk.
Examples include all surfaces with genus g>1 such as hyper-elliptic curves.
For every hyperbolic Riemann surface, the fundamental group is isomorphic to a Fuchsian group, and thus the surface can be modelled by a Fuchsian model H/Γ where H is the upper half-plane and Γ is the Fuchsian group. The set of representatives of the cosets of H/Γ are free regular sets and can be fashioned into metric fundamental polygons. Quotient structures as H/Γ are generalized to Shimura varieties.
Unlike elliptic and parabolic surfaces, no classification of the hyperbolic surfaces is possible. Any connected open strict subset of the plane gives a hyperbolic surface; consider the plane minus a Cantor set. A classification is possible for surfaces of finite type: those with finitely generated fundamental group. Any one of these has a finite number of moduli and so a finite dimensional Teichmüller space. The problem of moduli (solved by Lars Ahlfors and extended by Lipman Bers) was to justify Riemann's claim that for a closed surface of genus g, 3g - 3 complex parameters suffice.
When a hyperbolic surface is compact, then the total area of the surface is 4π(g-1), where g is the genus of the surface; the area is obtained by applying the Gauss-Bonnet theorem to the area of the fundamental polygon.
In art and literature
- One of M. C. Escher's works, Print Gallery, is laid out on a cyclically growing grid that has been described as a Riemann surface.
- In Aldous Huxley's novel Brave New World, "Riemann Surface Tennis" is a popular game.



![[\wp'(z)]^2=4[\wp(z)]^3-g_2\wp(z)-g_3,](../../images/157/15745.png)
