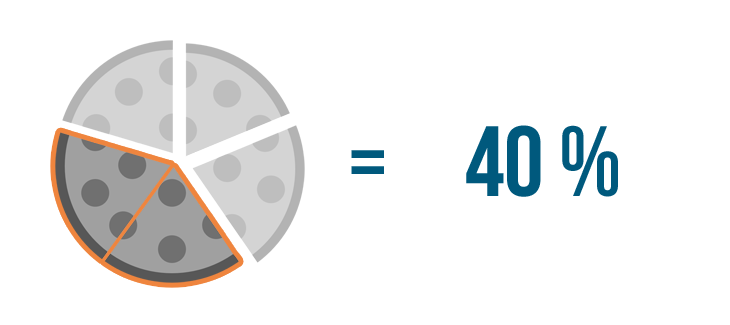Percents
Introduction to Percentages

What are percentages?
A percentage is another way of writing a decimal. Just like decimals, a percentage is a part of a whole . Basically, it's less than 1 whole thing, but more than 0 .
We use percentages all the time in real life. For example, have you ever left a fifteen percent tip at a restaurant? Or bought something on sale for twenty percent off? Those are both percentages— 15 percent and 20 percent .
Click through the slideshow to learn how percentages work.
Writing percentages
As you saw in the slideshow, every percentage has two parts: a number and the percent sign ( % ). When you write a percentage, you'll write the number first, then the percent sign. Let's try it! How would you write this percentage?
nine percent
First, we'll write the number, nine , and then the percent sign ( % ). So our percentage will look like this:
9%
Try This!
Try writing the correct percentage in the box.






Reading percentages
When you read a percentage out loud, you'll need to read two parts: the number and the percent sign ( % ). Let's look at an example:
25%
25% is twenty five out of one hundred . We'd read 25% like this:
twenty-five percent
Sometimes percentages might have a decimal . For example:
7.5%
Here, 7.5% means we have seven-and-a-half out of one hundred . We'd read it like this:
seven point five percent
OR
seven and a half percent
You can read any percentage with a decimal point like this. How about 10.25% ? That's ten and one quarter out of one hundred , so we'd read it as ten point two five percent , or ten and a quarter percent .
Try This!
Try reading each of the percentages below aloud.

Comparing percentages
Let's imagine you're shopping for apple juice. You find two different kinds—one contains 20% real juice, while the other contains 50% real juice.

Do you know which bottle has more real juice? Since both bottles are the same size, we can simply compare the numbers to see which percentage is larger .

50 is larger than 20 , so 50% is a larger percentage than 20% . The larger the number next to the percent sign, the larger the percentage.
What about these percentages?
7% and 17%
Which is larger? Again, we'll look to see which number is larger. 17 is larger than 7 , so 17% is a larger percentage than 7% .
Comparing percentages with decimals
What if you had to compare two percentages like this?
5.4% and 5.5%
At first glance, it might be difficult to tell which percentage is larger. Remember, this is just another way of asking, "Which is larger, five and four-tenths of a percent or five and five-tenths of a percent ?" Since the first number is the same for both fractions, we'll compare the numbers to the right of the decimal place.

5 is larger than 4 , so 5.5% is larger than 5.4% .
What about these percentages?
5.55% and 5.56%
Again, since the first number is the same, we'll compare the numbers to the right of the decimal place.

56 is larger than 55 , so 5.56% is larger than 5.55% .