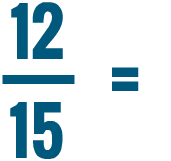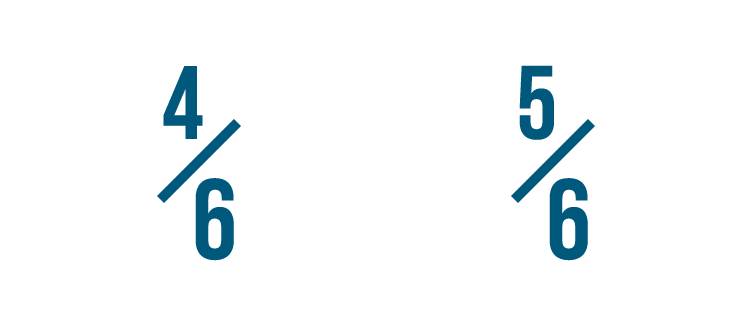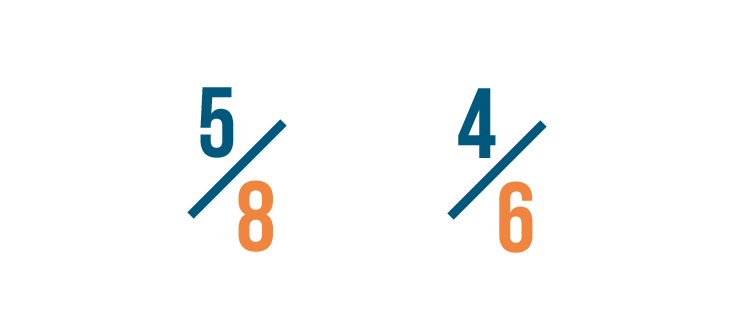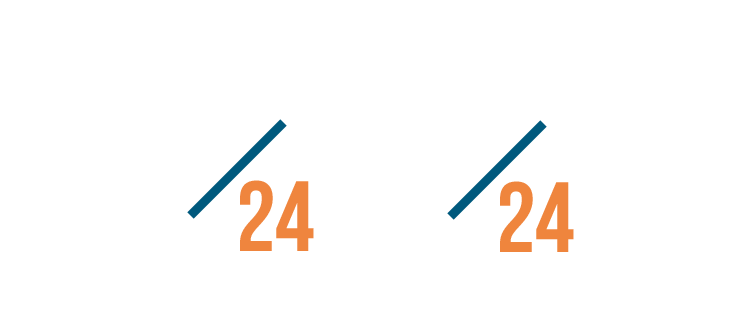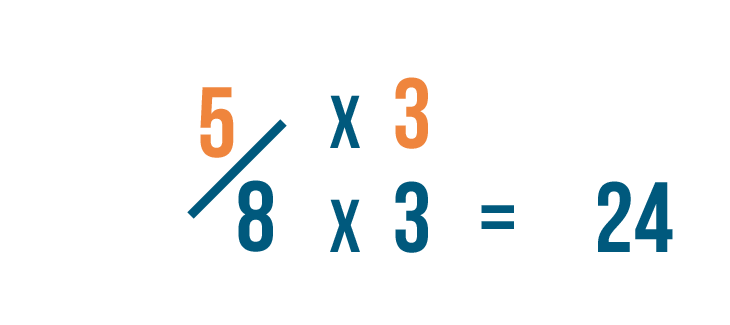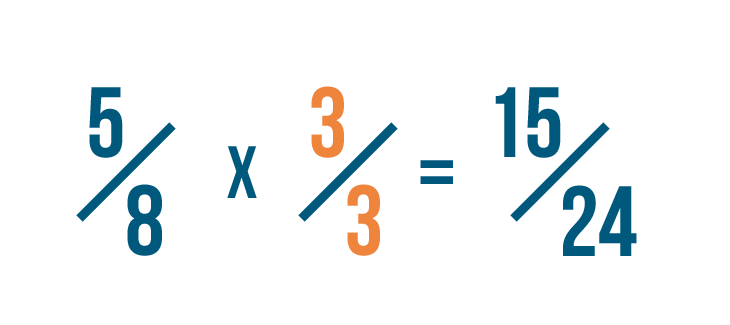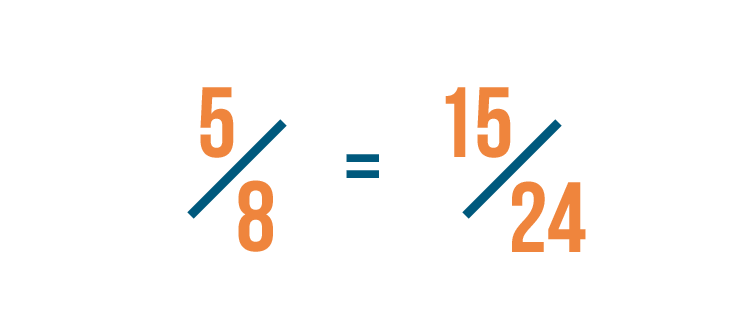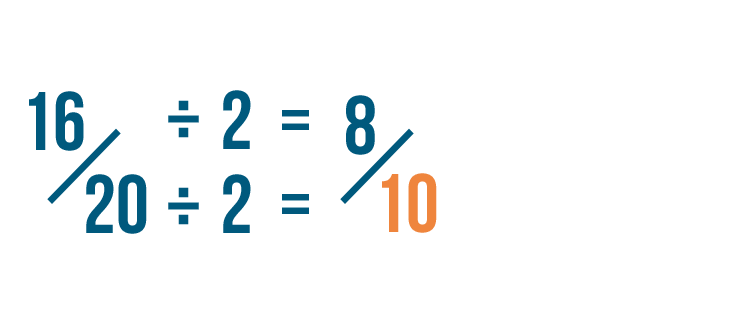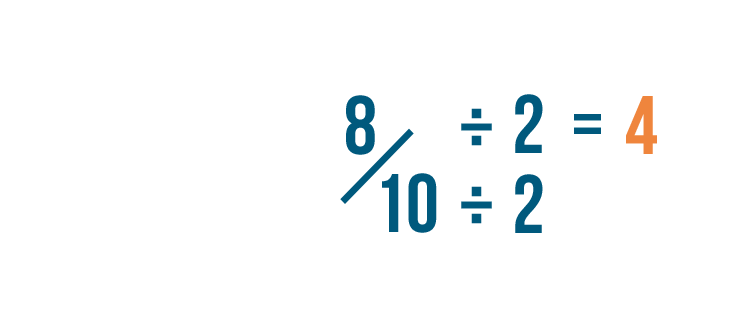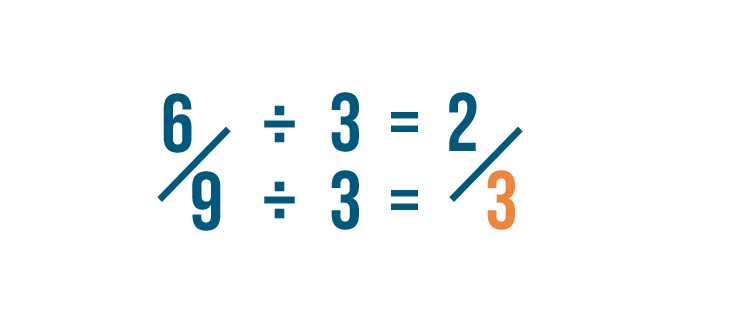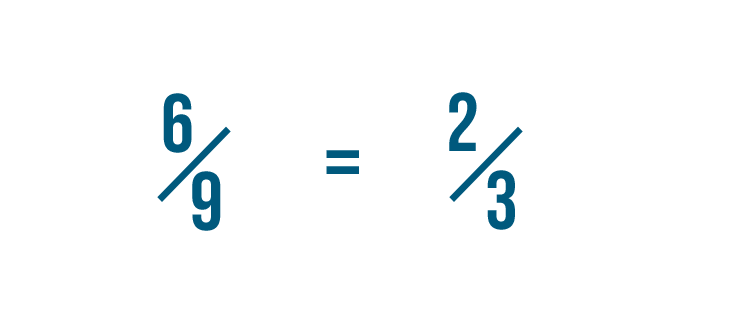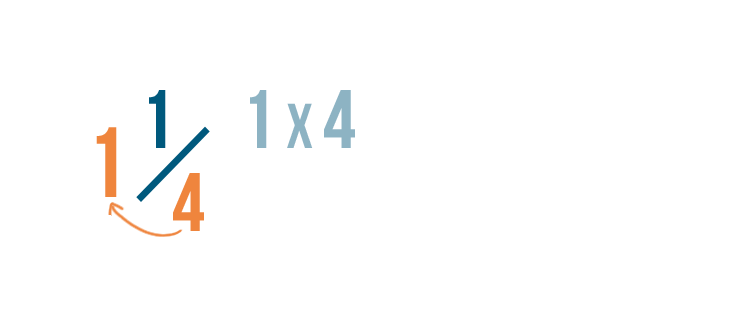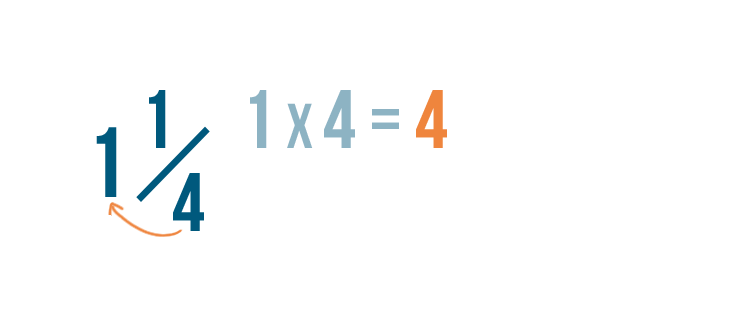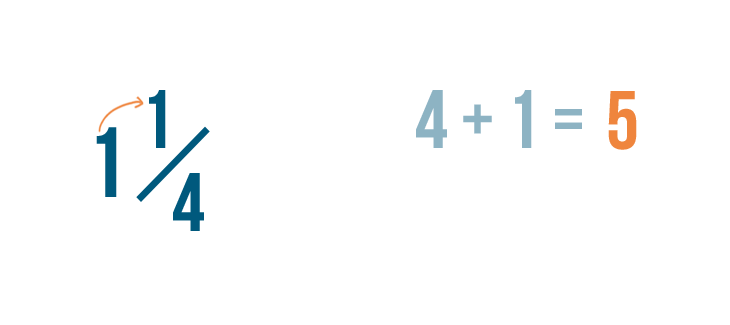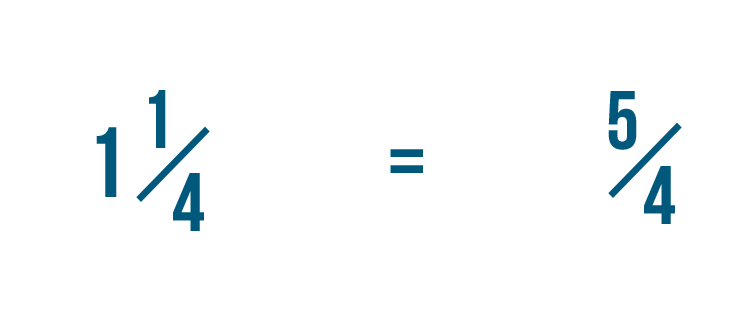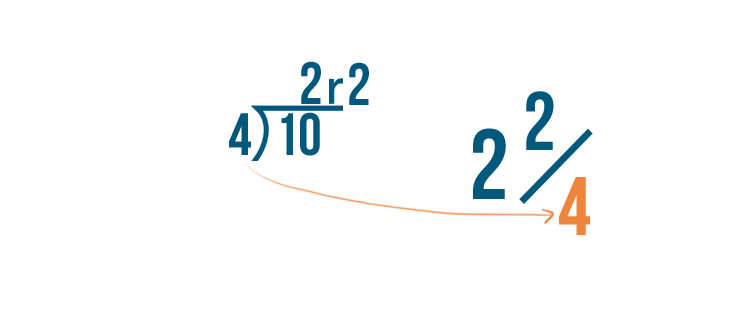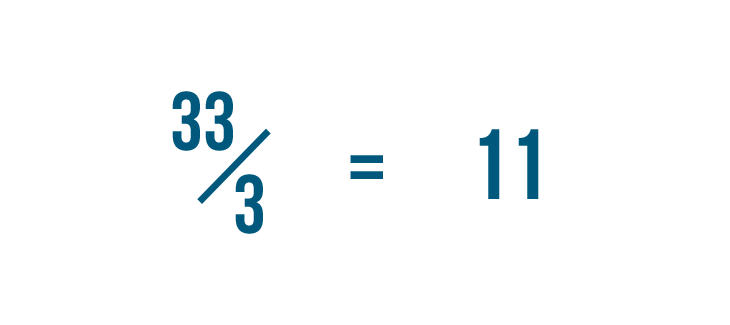Comparing fractions
In
Introduction to Fractions
, we learned that fractions are a way of showing
part
of something. Fractions are useful, since they let us tell exactly how much we have of something. Some fractions are larger than others. For example, which is larger:
6/8
of a pizza or
7/8
of a pizza?
In this image, we can see that
7/8
is larger. The illustration makes it easy to
compare
these fractions. But how could we have done it without the pictures?
Click through the slideshow to learn how to compare fractions.
-
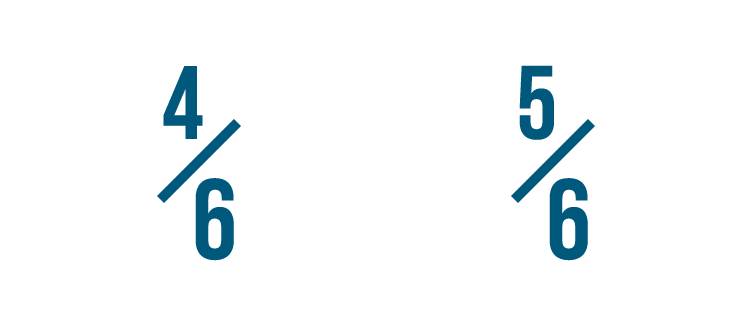
Earlier, we saw that fractions have two parts.
-

One part is the top number, or
numerator
.
-

The other is the bottom number, or
denominator
.
-

The denominator tells us how many
parts
are in a whole.
-

The numerator tells us how many of those parts we have.
-

When fractions have the same denominator, it means they're split into the same number of parts.
-

This means we can
compare
these fractions just by looking at the numerator.
-

Here,
5
is more than
4
...
-

Here,
5
is more than
4
...so we can tell that
5/6
is more than
4/6
.
-

Let's look at another example. Which of these is larger:
2/8
or
6/8
?
-

If you thought
6/8
was larger, you were right!
-

Both fractions have the same denominator.
-

So we compared the numerators.
6
is larger than
2
, so
6/8
is more than
2/8
.
-

As you saw, if two or more fractions have the same denominator, you can compare them by looking at their numerators. As you can see below,
3/4
is larger than
1/4
. The larger the numerator, the larger the fraction.
Comparing fractions with different denominators
On the previous page, we compared fractions that have the same
bottom numbers
, or
denominators
. But you know that fractions can have
any
number as a denominator. What happens when you need to compare fractions with different bottom numbers?
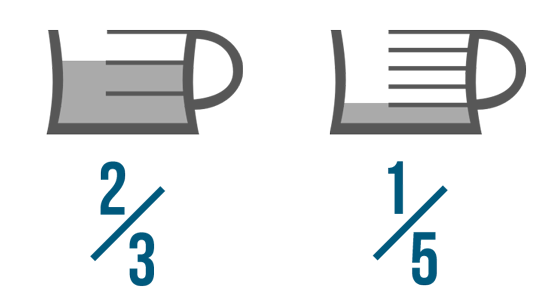
For example, which of these is larger:
2/3
or
1/5
? It's difficult to tell just by looking at them. After all,
2
is larger than
1
, but the denominators aren't the same.
If you look at the picture, though, the difference is clear:
2/3
is larger than
1/5
. With an illustration, it was easy to compare these fractions, but how could we have done it without the picture?
Click through the slideshow to learn how to compare fractions with different denominators.
-

Let's compare these fractions:
5/8
and
4/6
.
-

Before we compare them, we need to change both fractions so they have the same
denominator
, or bottom number.
-
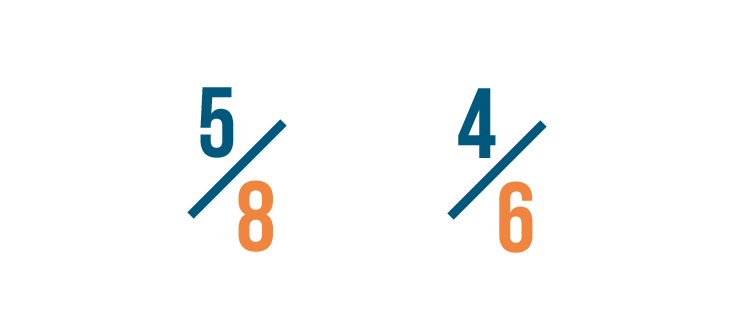
First, we'll find the smallest number that can be divided by both denominators. We call that the
lowest common denominator
.
-

Our first step is to find numbers that can be divided evenly by
8
.
-

Using a multiplication table makes this easy. All of the numbers on the
8
row can be divided evenly by
8
.
-

Now let's look at our second denominator:
6
.
-

We can use the multiplication table again. All of the numbers in the
6
row can be divided evenly by
6
.
-

Let's compare the two rows. It looks like there are a few numbers that can be divided evenly by both
6
and
8
.
-

24
is the smallest number that appears on both rows, so it's the
lowest common denominator
.
-
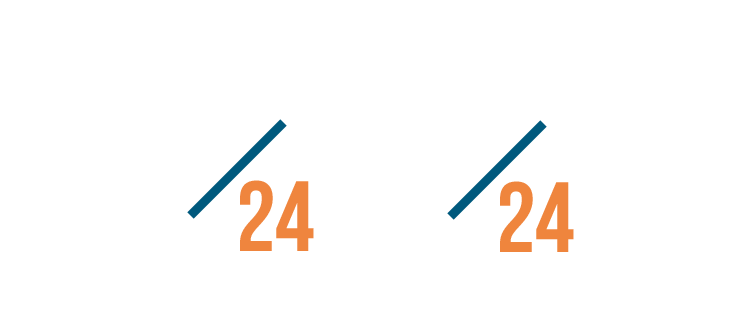
Now we're going to change our fractions so they both have the same denominator:
24
.
-

To do that, we'll have to change the numerators the same way we changed the denominators.
-

Let’s look at
5/8
again. In order to change the denominator to
24
...
-

Let’s look at
5/8
again. In order to change the denominator to
24
...we had to multiply
8
by
3
.
-
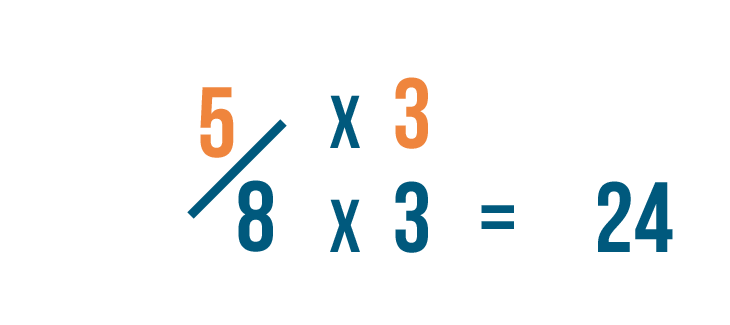
Since we multiplied the denominator by
3
, we'll also multiply the numerator, or top number, by
3
.
-

5
times
3
equals
15
. So we've changed
5/8
into
15/24
.
-

We can do that because any number over itself is equal to
1
.
-
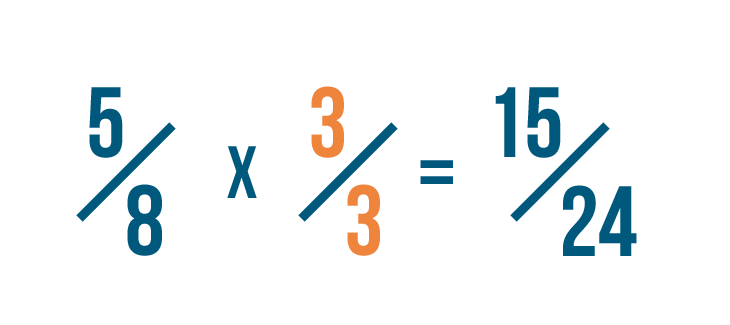
So when we multiply
5/8
by
3/3
...
-

So when we multiply
5/8
by
3/3
...we're really multiplying
5/8
by
1
.
-

Since any number times
1
is equal to itself...
-
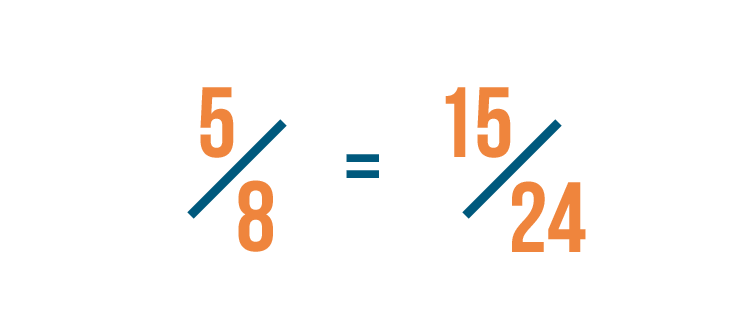
Since any number times
1
is equal to itself...we can say that
5/8
is equal to
15/24
.
-

Now we'll do the same to our other fraction:
4/6
. We also changed its denominator to
24
.
-

Our old denominator was
6
. To get
24
, we multiplied
6
by
4
.
-

So we'll also multiply the numerator by
4
.
-

4
times
4
is
16
. So
4/6
is equal to
16/24
.
-

Now that the denominators are the same, we can compare the two fractions by looking at their numerators.
-

16/24
is larger than
15/24
...
-

16/24
is larger than
15/24
... so
4/6
is larger than
5/8
.
-

Reducing fractions
Which of these is larger:
4/8
or
1/2?
If you did the math or even just looked at the picture, you might have been able to tell that they're
equal
. In other words,
4/8
and
1/2
mean the same thing, even though they're written differently.
If
4/8
means the same thing as
1/2
, why not just call it that?
One-half
is easier to say than
four-eighths
, and for most people it's also easier to understand. After all, when you eat out with a friend, you split the bill in
half
, not in
eighths
.
If you write
4/8
as
1/2
, you're
reducing
it. When we
reduce
a fraction, we're writing it in a simpler form. Reduced fractions are always
equal
to the original fraction.
We already reduced
4/8
to
1/2
. If you look at the examples below, you can see that other numbers can be reduced to
1/2
as well. These fractions are all
equal
.
5/10 = 1/2
11/22 = 1/2
36/72 = 1/2
These fractions have all been reduced to a simpler form as well.
4/12 = 1/3
14/21 = 2/3
35/50 = 7/10
Click through the slideshow to learn how to reduce fractions by
dividing
.
-

Let's try reducing this fraction:
16/20
.
-

Since the numerator and denominator are
even numbers
, you can divide them by
2
to reduce the fraction.
-

First, we'll divide the numerator by
2
.
16
divided by
2
is
8
.
-
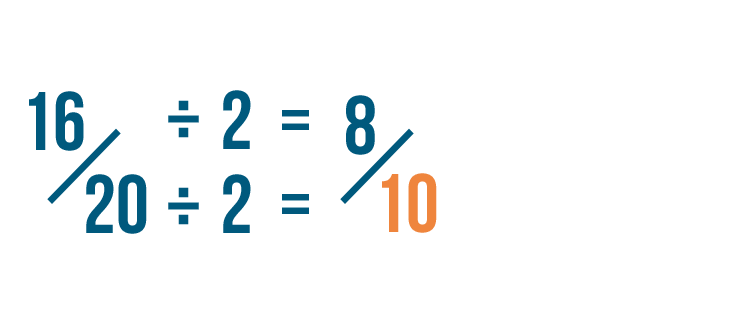
Next, we'll divide the denominator by
2
.
20
divided by
2
is
10
.
-

We've reduced
16/20
to
8/10
. We could also say that
16/20
is equal to
8/10
.
-

If the numerator and denominator can still be divided by
2
, we can continue reducing the fraction.
-
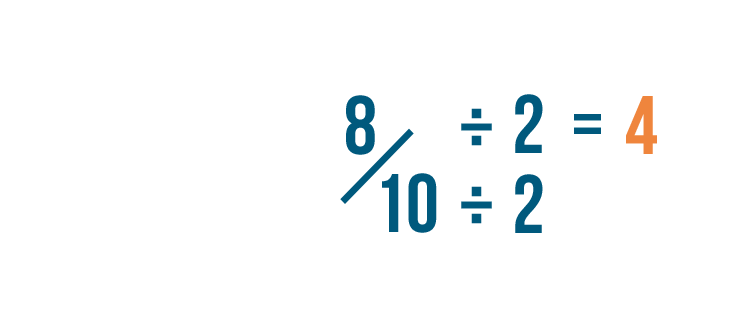
8
divided by
2
is
4
.
-

10
divided by
2
is
5
.
-

Since there's no number that
4
and
5
can be divided by, we can't reduce
4/5
any further.
-

This means
4/5
is the
simplest
form
of
16/20
.
-

Let's try reducing another fraction:
6/9
.
-

While the numerator is even, the denominator is an
odd number
, so we can't reduce by dividing by
2
.
-

Instead, we'll need to find a number that
6
and
9
can be divided by. A multiplication table will make that number easy to find.
-
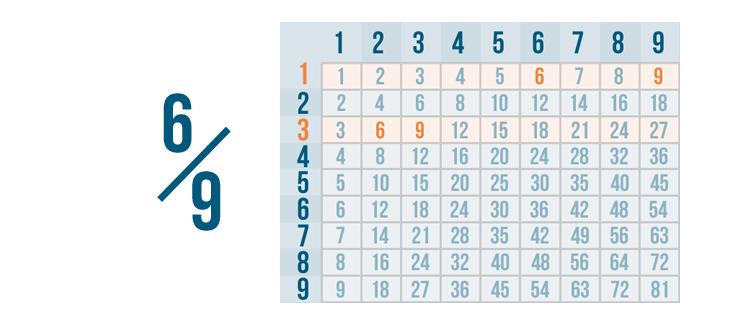
Let's find
6
and
9
on the
same
row
. As you can see,
6
and
9
can both be divided by
1
and
3
.
-

Dividing by
1
won't change these fractions, so we'll use the
largest
number that
6
and
9
can be divided by.
-
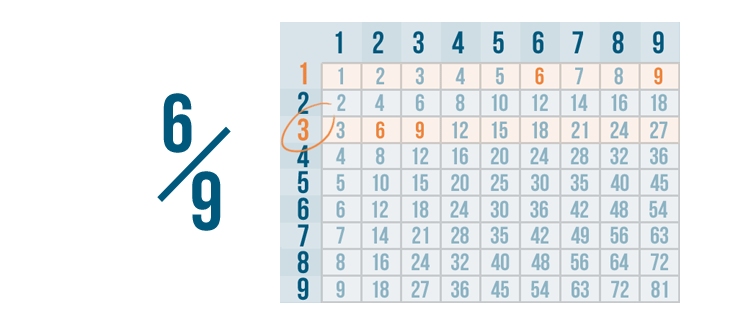
That's
3
. This is called the
greatest common divisor
, or
GCD
. (You can also call it the
greatest common factor
, or
GCF
.)
-
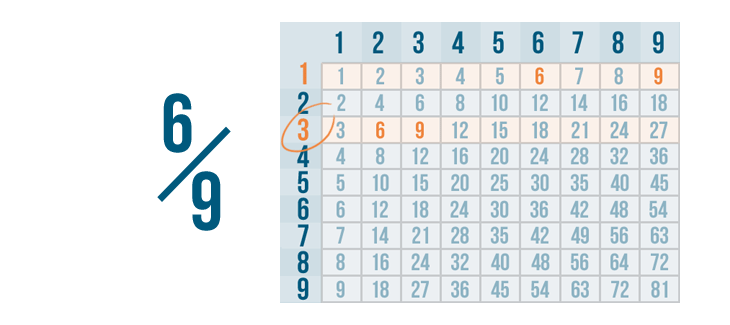
3
is the
GCD
of
6
and
9
because it's the
largest
number they can be divided by.
-

So we'll divide the numerator by
3
.
6
divided by
3
is
2
.
-
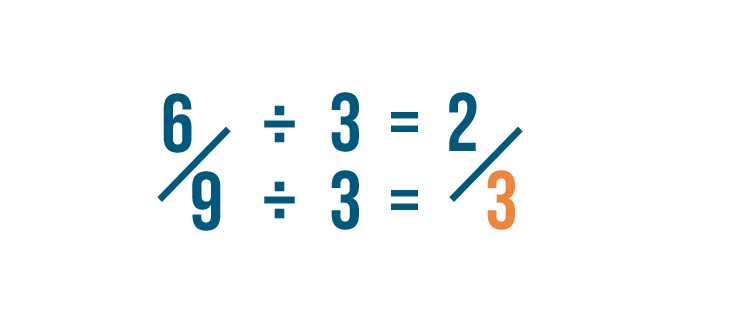
Then we'll divide the denominator by
3
.
9
divided by
3
is
3
.
-
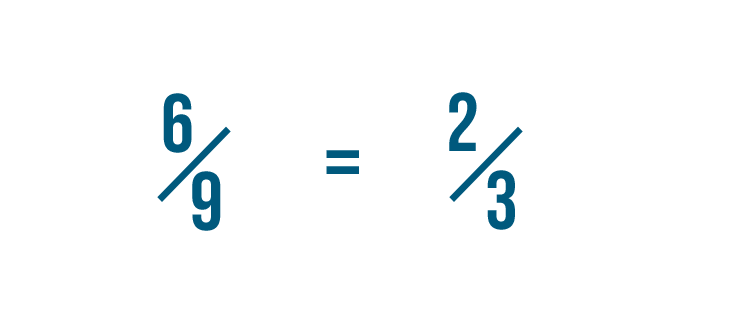
Now we've reduced
6/9
to
2/3
, which is its simplest form. We could also say that
6/9
is equal to
2/3
.
-

Irreducible fractions
Not all fractions can be reduced. Some are already as simple as they can be. For example, you can't reduce
1/2
because there's no number other than
1
that both
1
and
2
can be divided by. (For that reason, you can't reduce
any
fraction that has a
numerator
of
1
.)
Some fractions that have larger numbers can't be reduced either. For instance,
17/36
can't be reduced because there's no number that both
17
and
36
can be divided by. If you can't find any
common multiples
for the numbers in a fraction, chances are it's
irreducible
.
Try This!
Reduce each fraction to its simplest form.
Mixed numbers and improper fractions
In the
previous lesson
, you learned about
mixed numbers
. A mixed number has both a
fraction
and a
whole number
. An example is
1 2/3
. You'd read
1 2/3
like this:
one and two-thirds
.
Another way to write this would be
5/3
, or
five-thirds
. These two numbers look different, but they're actually the same.
5/3
is an
improper fraction
. This just means the numerator is
larger
than the denominator.
There are times when you may prefer to use an improper fraction instead of a mixed number. It's easy to change a mixed number into an improper fraction. Let's learn how:
-

Let's convert
1 1/4
into an improper fraction.
-

First, we'll need to find out how many
parts
make up the whole number:
1
in this example.
-
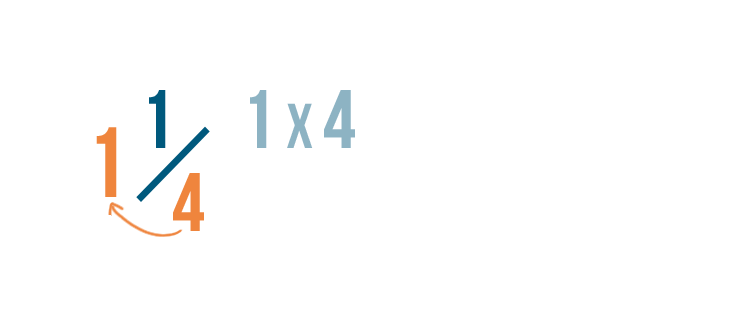
To do this, we'll multiply the
whole number
,
1
, by the denominator,
4
.
-
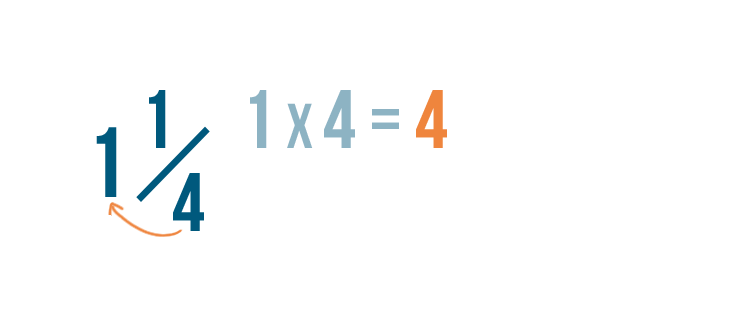
1
times
4
equals
4
.
-

Now, let's add that number,
4
, to the numerator,
1
.
-
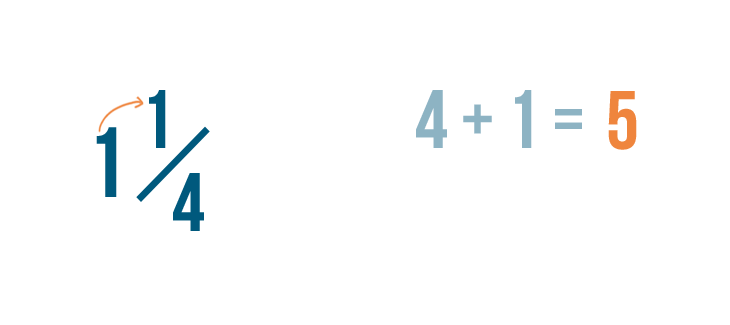
4
plus
1
equals
5
.
-
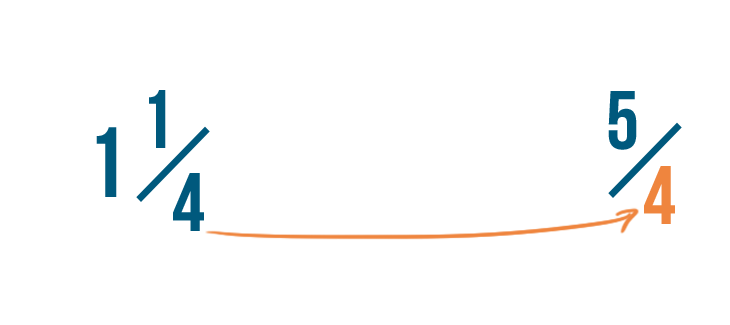
The denominator stays the same.
-
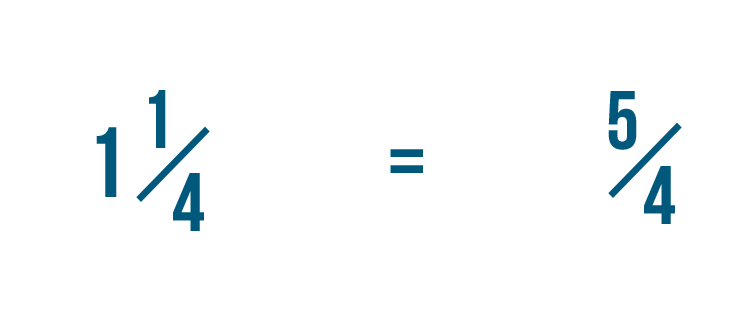
Our improper fraction is
5/4,
or
five-fourths
. So we could say that
1 1/4
is equal to
5/4
.
-

This means there are
five
1/4
s in
1 1/4
.
-

Let's convert another mixed number:
2 2/5
.
-

First, we'll multiply the whole number by the denominator.
2
times
5
equals
10
.
-

Next, we'll add
10
to the numerator.
10
plus
2
equals
12
.
-

As always, the denominator will stay the same.
-

So
2 2/5
is equal to
12/5
.
-

Try This!
Try converting these mixed numbers into improper fractions.
Converting improper fractions into mixed numbers
Improper fractions are useful for math problems that use fractions, as you'll learn later. However, they're also more difficult to read and understand than
mixed
numbers
. For example, it's a lot easier to picture
2 4/7
in your head than
18/7
.
Click through the slideshow to learn how to change an improper fraction into a mixed number.
-

Let's turn
10/4
into a mixed number.
-

You can think of any fraction as a
division
problem
. Just treat the line between the numbers like a division sign (
/
).
-

So we'll
divide
the numerator,
10
, by the denominator,
4
.
-

10
divided by
4
equals
2
...
-

10
divided by
4
equals
2
... with a remainder of
2
.
-

The answer,
2,
will become our whole number because
10
can be divided by
4
twice
.
-

And the
remainder
,
2
, will become the numerator of the fraction because we have
2
parts left over.
-
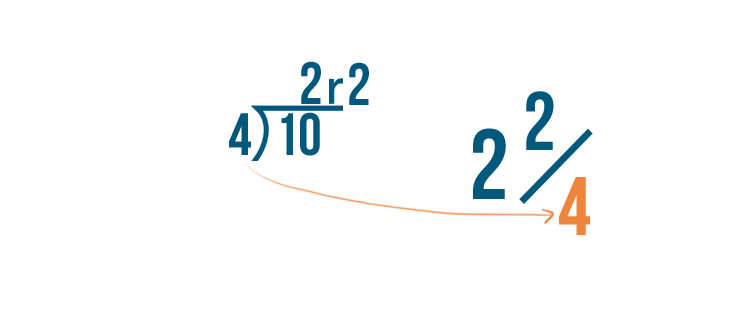
The denominator remains the same.
-

So
10/4
equals
2 2/4
.
-

Let's try another example:
33/3
.
-

We'll divide the numerator,
33
, by the denominator,
3
.
-

33
divided by
3
...
-

33
divided by
3
... equals
11,
with no remainder.
-

The answer,
11,
will become our whole number.
-
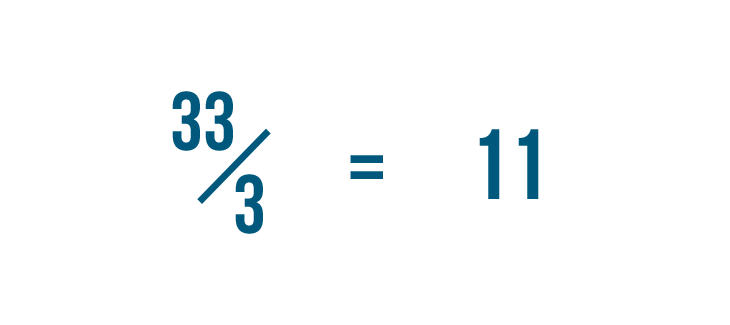
There is no remainder, so we can see that our improper fraction was actually a whole number.
33/3
equals
11
.
-

Try This!
Try converting these improper fractions into mixed numbers.