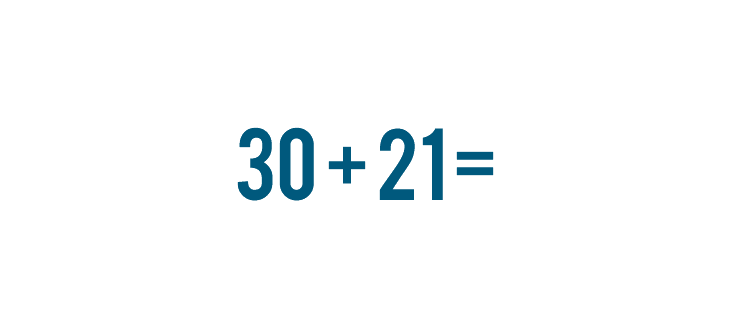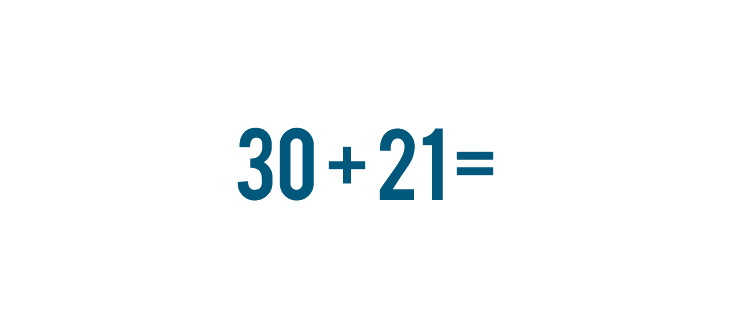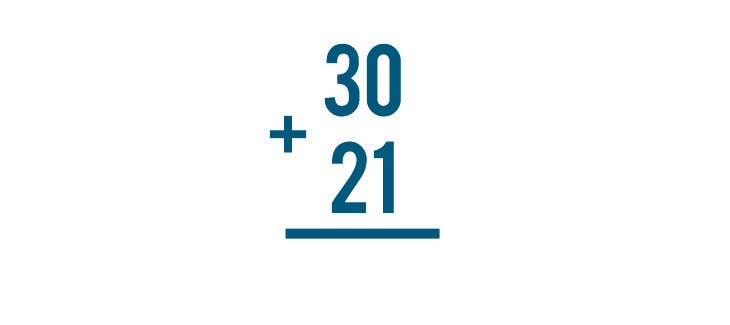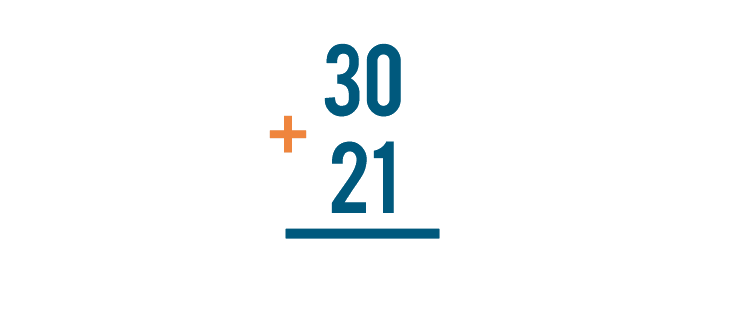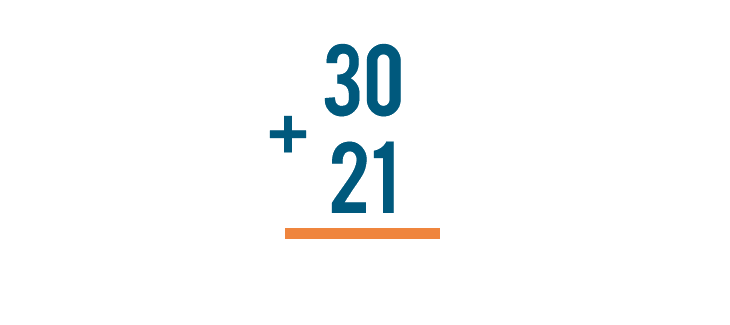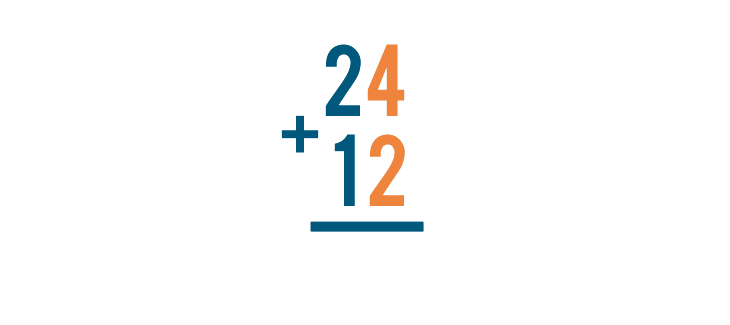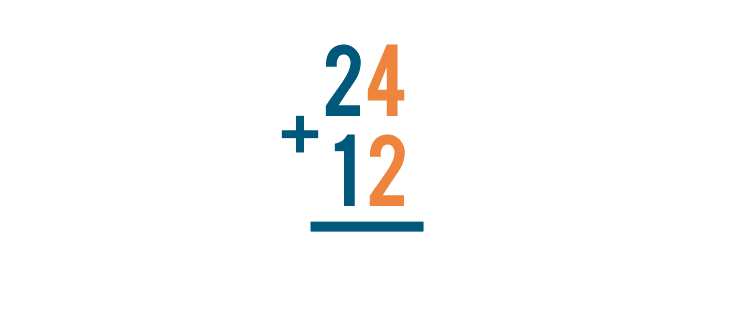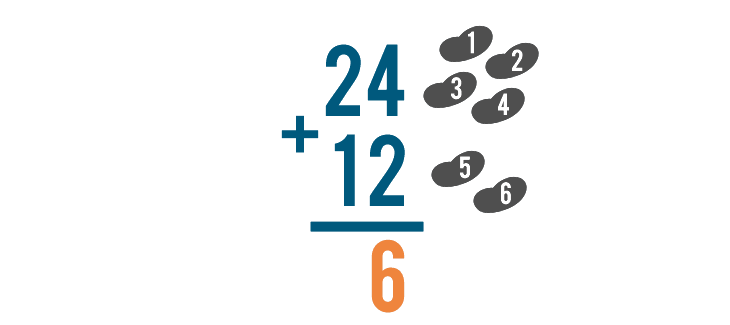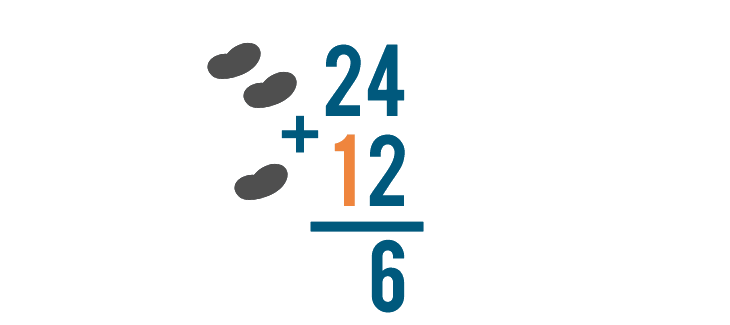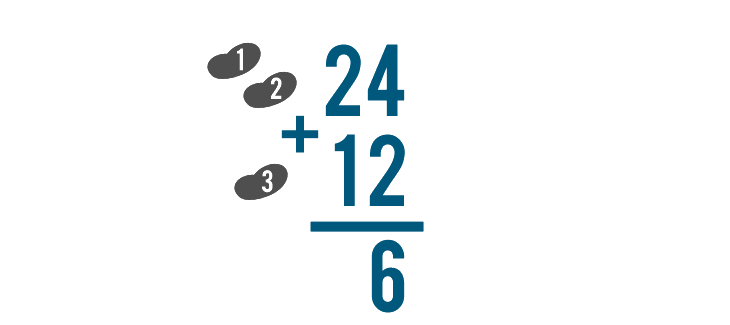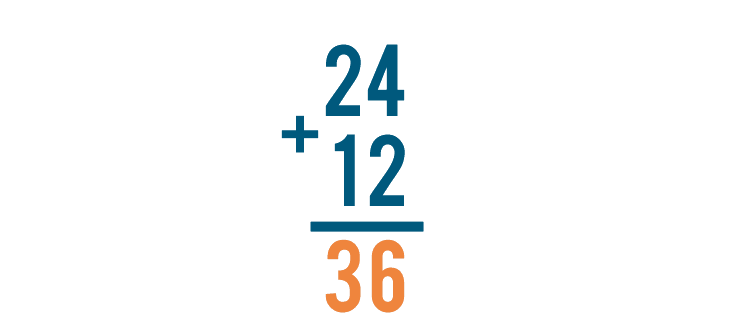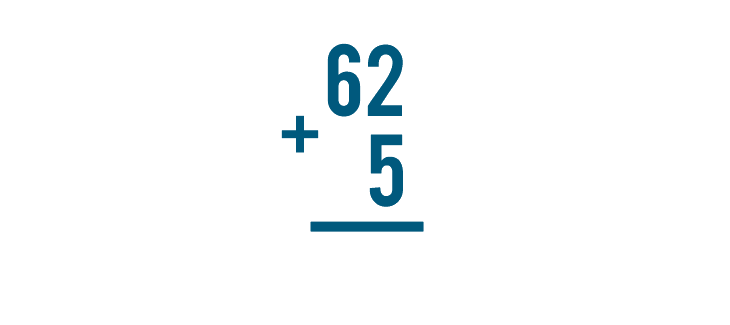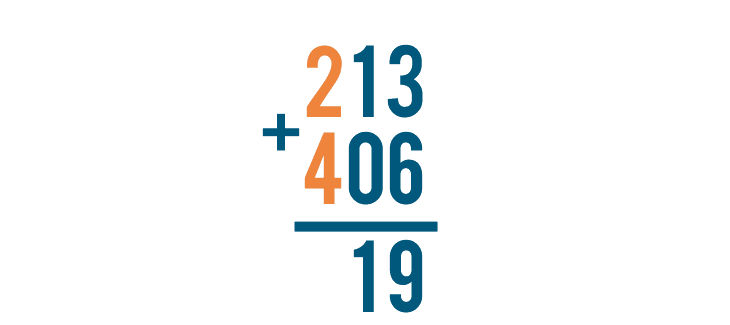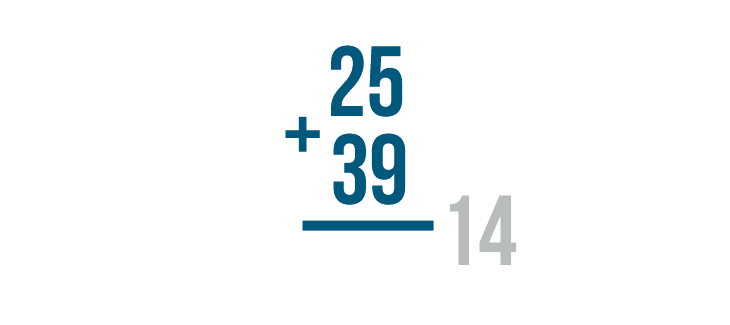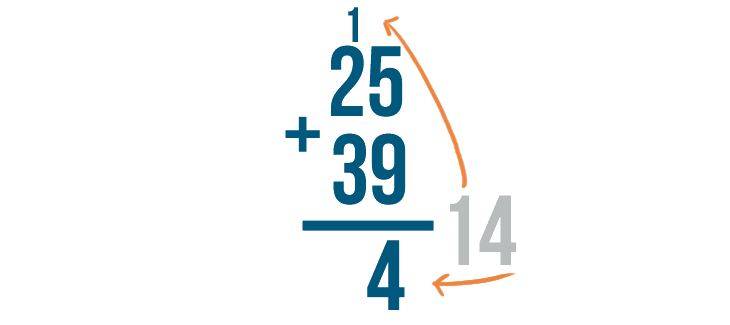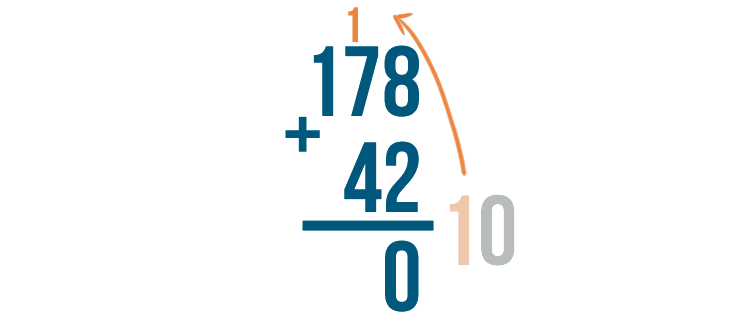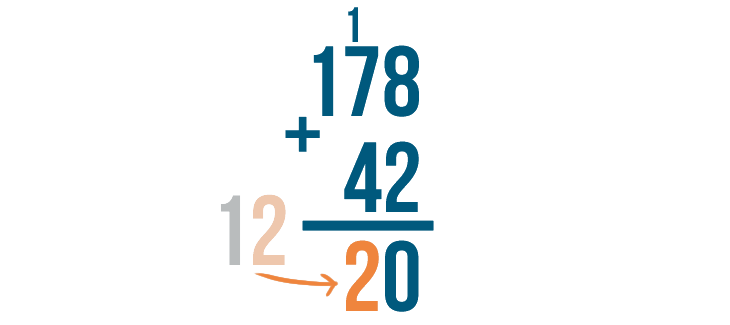Adding larger numbers
As we saw in
Introduction to Addition
, you can often use
counting
and
visuals
to solve basic addition problems. For instance, imagine that
3
people are going on a trip and
2
more decided to join. To find out how many people were going total, you could represent the situation like this:
Once you look at the problem visually, you can count and see that
5
people are going on the trip.
What if you have a bigger problem to solve? Imagine that a few groups of people are going somewhere together.
30
people travel on one bus, and
21
travel on another. We could write this as
30 + 21
.
It might not be a good idea to solve this problem by counting. First of all, no matter how you choose to count, it would probably take a pretty long time to set up the problem. Imagine drawing 30 and 21 pencil marks on the page, or counting out that many little objects! Second, actually counting the objects could take long enough that you might even lose track.
For this reason, when people solve a large addition problem, they set up the problem in a way that makes it easier to solve one step at a time. Let's look at the problem we discussed above,
30 + 21
.
-
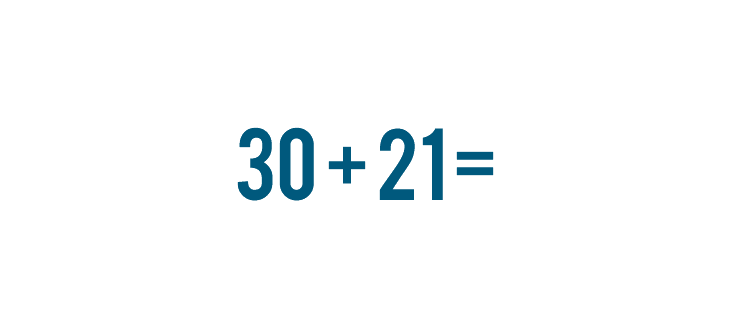
In the last lesson, we learned how to write expressions. However, when we're adding
larger numbers
, it helps to write the expressions in a different way.
-
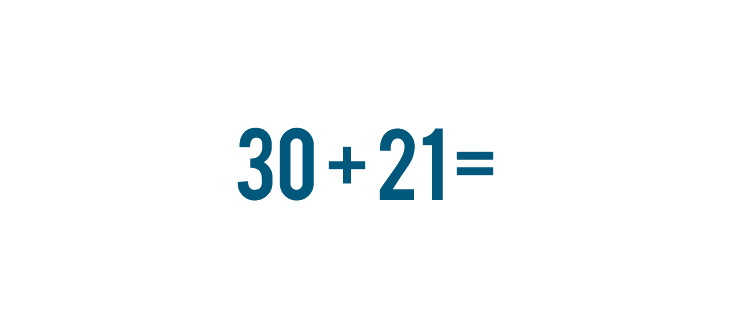
Instead of writing the numbers side by side...
-
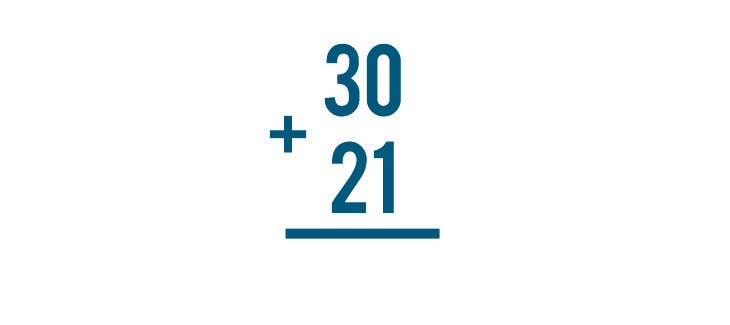
...We
stack
them on top of one another.
-
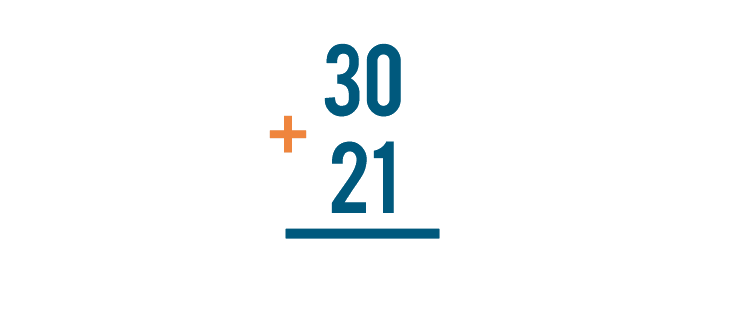
Put the
plus sign
(
+
) to the
left
of the numbers.
-
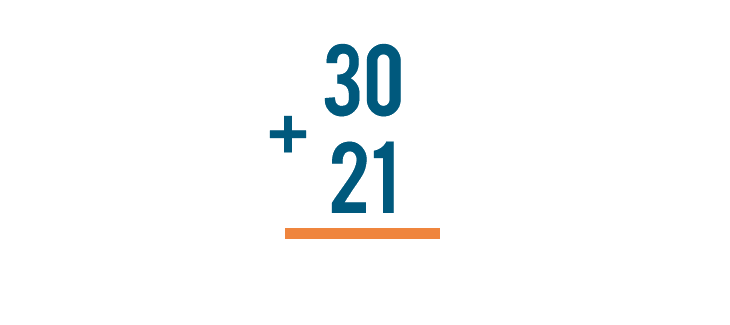
Instead of an
equals
sign
(
=
), put a
line
underneath the lower number.
-

When you
stack
an addition expression, make sure the numbers are lined up correctly. The numbers should always be lined up on the
right
.
-

Let's look at another problem,
365 + 1
. See how the
1
is under the
5
?
-

No matter how large the numbers you're adding are, the
digit
s on the
right
should always be lined up.
-

We can see that
30 + 21
and
 mean the same thing. They're just written differently.
mean the same thing. They're just written differently.
Solving Stacked Addition Problems
To solve stacked addition problems, all you need are the skills you learned in
Introduction to Addition
.
-

Let's try solving this problem,
24 + 12
.
-
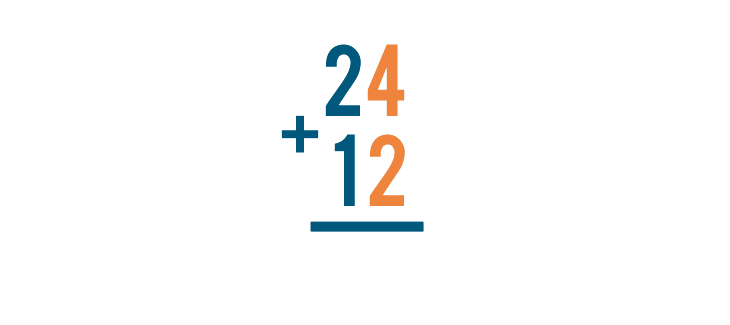
To solve a stacked addition problem, start by adding the digits farthest to the
right
.
-
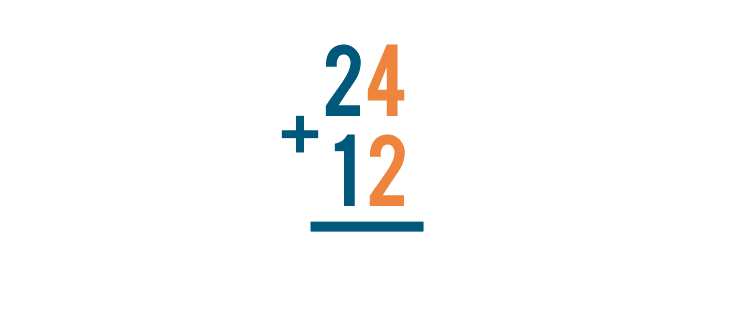
In this case, that means we'll add
4
and
2
.
-

Like with any addition problem, we can use
counting
to help us add. Since our first digit is
4
, we'll start with four objects.
-

We're adding 4 to
2
, so we'll use two more objects.
-

We can count to get a total of
6
. We know that
4 + 2 = 6
.
-
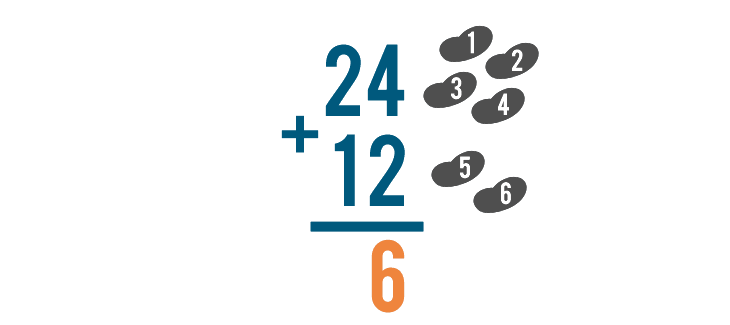
Write
6
under the line. It's important to put it directly
beneath
the numbers we just added.
-

Next, we'll add the digits to the
left
of the ones we just added. That's
2
and
1
.
-

We'll use two objects to show the
2
.
-
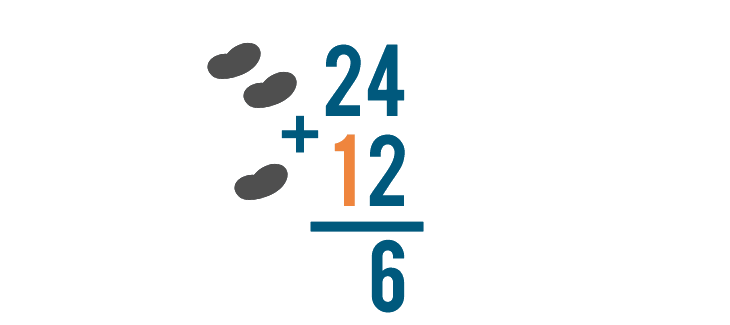
Next, we'll add one object to represent the
1
.
-
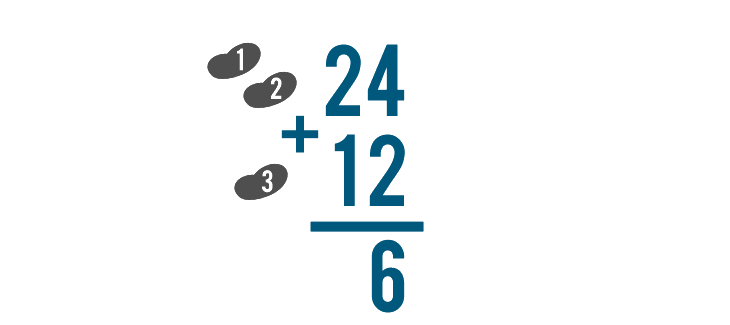
When we count, we get
3
.
-

We'll write the
3
under the line, beneath the
2
and
1
.
-
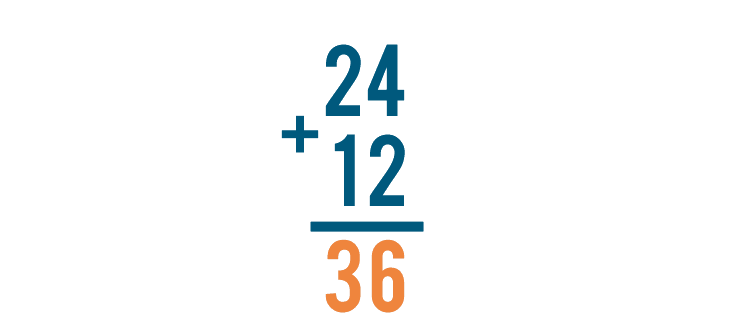
We're done! Our total is
36
, or thirty-six.
-
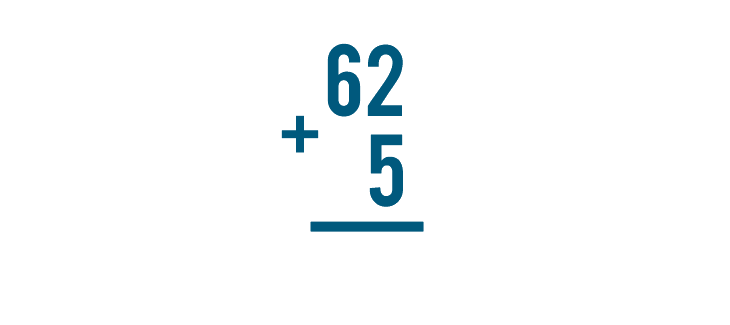
Let's look at one more problem, just for practice. This time, we'll solve
62 + 5
.
-

We'll start by adding the digits on the right,
2
and
5
.
-

2 + 5
is
7
.
-

Now, let's add the digits to the left. The top digit is
6
, but there's nothing beneath it.
-

6
plus nothing is
6
, so we'll write a
6
below the line.
-

Our total is
67
, or sixty-seven.
-

As you saw, stacked addition problems are always solved from
right
to left
. This means that you always start by adding the digits on the
right
.
Try This!
Solve the addition problems below. Then, check your answer by typing it into the box.
Adding Very Large Numbers
Stacked addition can also be used for adding
larger numbers
. No matter how many digits are in the numbers you're adding, you add them the same way: from right to left.
-

Let's try adding two
3-digit
numbers. We'll complete this expression:
213 + 406
.
-

As always, we start with the digits on the
far right
. Here, that means we'll solve
3 + 6
.
-

3 + 6
is
9
, so we'll write
9
directly beneath the
3
and
6
.
-

Next, we'll add the digits to the
left
of the ones we just added,
1
and
0
.
-

1 + 0
is
1
, so we'll write
1
below the line. Make sure to put it
directly beneath
the digits we just added, to the
left
of the
9
.
-
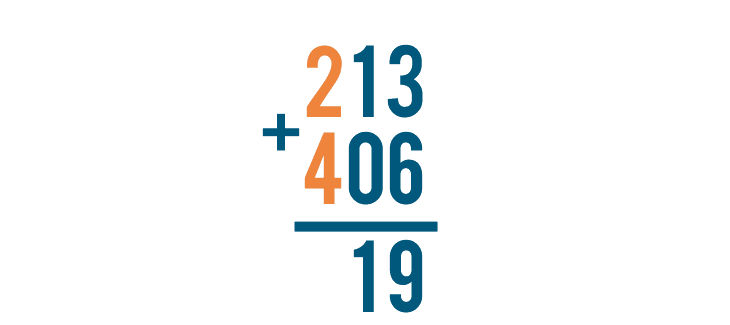
Finally, let's add the next set of digits,
2
and
4
.
-

2 + 4
is
6
. Let's write the
6
beneath the line, under the
2
and
4
.
-

The problem is complete. Our total is
619
, or six hundred nineteen.
213 + 406 = 619
.
-

Try This!
Add these large numbers. Then, check your answer by typing it in the box.
Using Carrying
On the last page, you practiced adding vertically stacked numbers. Some problems need an extra step. For example, let's look at the following problem:
Our first step is to add the digits on the right—
5
and
9
. However, you might notice there isn't room to write the sum,
14
. When the sum of two digits in a math problem is
greater than 9
, the normal way of adding stacked numbers won't work. You'll have to use a technique called
carrying
.
-

Let's see how it works. We'll try this problem,
25 + 39
.
-

As usual, we'll start by adding the digits on the
right
. Here, that's
5
and
9
.
-
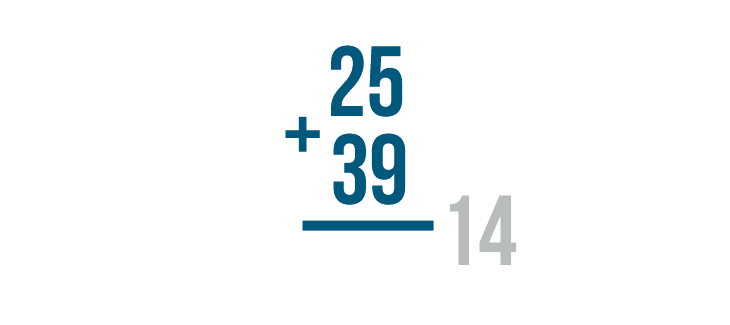
5 + 9
is
14
, but there's no room to write both digits in
14
underneath the
5
and
9
.
-

We'll write the right digit,
4
, under the line...
-

We'll write the right digit,
4
, under the line... then we'll
carry
the left digit,
1
, up to the next set of digits in the problem.
-
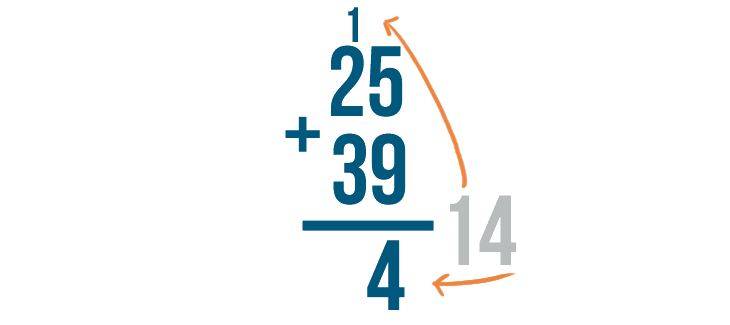
Do you see what we did? Our sum was
14
. We put the
4
underneath the line, and carried the
1
and placed it above the next set of digits.
-

Next, we'll add the left digits. Since we carried the
1
, we'll add it too.
-

1 + 2 + 3
is
6
, so we'll write
6
below the line.
-

We're done.
25 + 39 = 64
.
-

Let's practice with one more problem:
178 + 42
.
-

As always, start by adding the digits on the right. Here, that's
8 + 2
.
-

8 + 2
is
10
, so it looks like we'll have to carry.
-

The
0
stays underneath the
8
and the
2
.
-
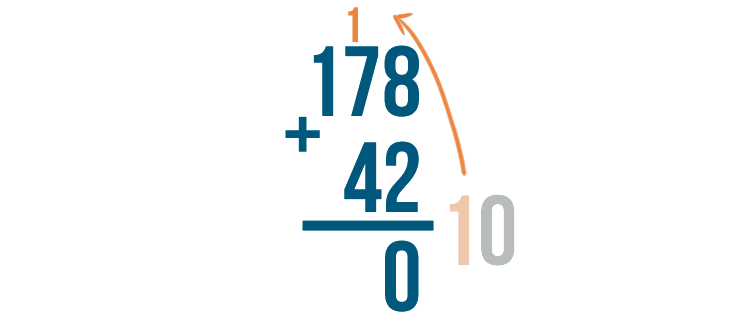
Carry the
1
and place it above the next set of digits to the left.
-

Now move left to add the next set of digits. Since we carried the
1
, add it too.
-

1 + 7 + 4
=
12
.
-
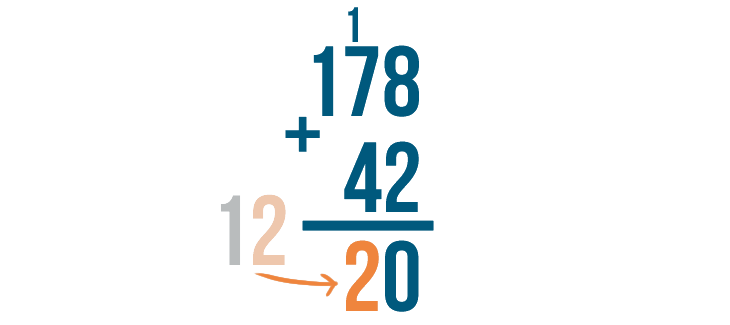
Put the right number,
2
, under the digits we added.
-

Carry the
1
and place it above the next column to the left.
-

To finish, add this column. Remember to include the
1
we just carried.
-

1 + 1 + nothing
is
2
, so we'll write
2
underneath the
1
.
-

We're done. Our answer is
220
.
178 + 42 = 220
.
-

As you carry, be careful to keep track of the various numbers. If you're writing problems down, be sure to write the carried digits in small print above the column of digits to the left.
Try This!
Solve these problems by carrying. Then, check your answer by typing it in the box.
Practice!
Practice adding these problems. You'll have to use carrying to solve some of the problems. There are
4
sets of problems with
3
problems each.
Set 1
Set 2
Set 3
Set 4


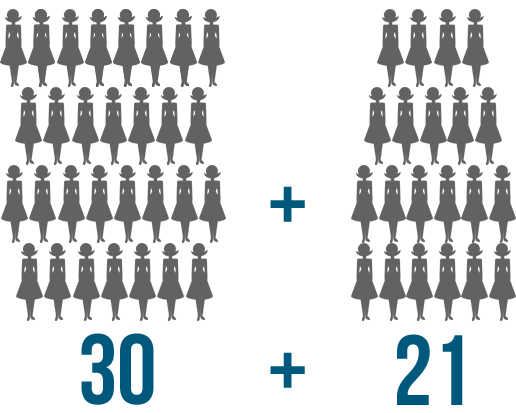
 mean the same thing. They're just written differently.
mean the same thing. They're just written differently.